

Christopher Sage’s works explores the various frameworks and foundations that influence and model our comprehension, from language to science, philosophy and theology, and how governing systems give rise to select patterns of thought. Playing with cognitive and optical shifts, Sage’s works merge disparate ideas ranging from religion and string theory, to language and art history. Contrasting concepts and opposing visual elements share a common space, were they threaten to brake apart as they intersect one another; and contradictions become suspended in a visual unity.
Mathematical systems govern the formation of Sage’s work, as the laws of physics govern the physical world. Sage employs Prime numbers to impact his compositions and their proportional relations. This mathematical underpinning leads the evolution of formal and aesthetic outcomes. While aesthetic principles of classical Golden section geometry stems from natural forms, Sage takes from patterns within our numeric system itself. Sage explains “I'm interested in Primes as a byproduct of an ordered, logical system. Their unique, concrete indivisibility, sits at odds with their erratic behaviour, appearing at unpredictable, seemingly random intervals.” It is perhaps not surprising to find various Prime numbers have been loaded by different cultures with superstitious or religious significances.
Christopher Sage's 'Glyphic forms' series is a play on the fundamental building blocks of language and comprehension. Drawing inspiration form various signs and symbols, Sage generates looped graphics that contradict laws of Euclidean geometry. The black and white printed forms play host to multiple recognisable signs fused together, with ambiguous spacial readings, akin to a Necker cube. This simultaneity leads to grey shades of understanding and shifting re-interpretations. Sage reflects on ways letters and other symbols are in a state of uncertainty, representing acoustical possibilities as they undergo changes between different regional or cultural pronunciations. He draws parallels between the manner we combine sounds to assemble words and meaning, with String theory's notions of multi-dimensional, resonating strings as the fundamental building blocks of the physical world. Sage's ambiguous alphabet offers a multi-perspective view point where the forms are in a state of constant flux.
The artworks of Christopher Sage are a prime example of clarity and comprehensibility - and yet they are full of mystery and contradictions. The basically constructivist works follow the rationality of mathematics, physics and geometry - and at the same time they are a document of the irrational, almost mystical side of these sciences. What we have here is kind of an irrational constructivism or surreal rationalism - a fact that contributes decisively to the cheerfulness of these works.
His paintings, says Christopher Sage tongue-in-cheek, "are in a kind of neurosis", a phrase that echoes the subtle irony of a typical English gentleman. It should be remembered, though, that good manners and correctness often have their counterpart in a desire for the absurd, even for cruel destruction. "Off with their heads!", demands the Queen of Hearts in the fantasy tale "Alice in Wonderland", written by a mathematician and gentleman educated in Oxford...
Constructivism is the most rational of all art forms, it is based on exact planning and measurement, precisely defined lines and planes. This also applies to the works of Christopher Sage. His pictorial spaces are interspersed with ribbons and threads that seem like units of measurement. Contrasts are created by open swirls of colour, which are set against clearly defined areas, just as plain surfaces lie next to painterly-structured ones. In terms of colour, bright tones stand next to rather dark and matt shades, shrill neon shines alongside subdued earthy hues.
And these contrasts grow into veritable dangers: The swirls of paint can create a devouring maelstrom or wind like insidious snakes, which - as in the antique marble group of Laocoon and his sons - bring agonising death. The sculpture String thing balances so delicately on its base that it threatens to fall down in the next moment. And we could just disappear under the wizard’s magic cloth, or - the more appropriate fate - plunge through Alice's mirror into a world of wonders. It is the unexpected that stirs up this fine-tuned constructivism. Mind you, there might hide a Jack the Ripper in the gentle English gentleman...
Whereas earlier works deal with such wondrous subjects as magicians, fortune-tellers and telekinetics, who can move objects by thought-work alone, the newer pieces mainly refer to the oddities and irrationalities of the "rational" sciences. Let's take three examples, three aspects that appear more frequently in the work.
Sage's paintings are constructed on the basis of prime numbers. Their principle is a clear, logical system based on indivisibility. And yet they exhibit erratic behaviour and are full of mystery. They appear at unpredictable, seemingly random intervals on the number line; we are far from knowing their exact quantity, all we know is that the series never ends, recent research has led to a number with 22,338,618 decimal places. Many consider the prime numbers to be the finest that mathematics has to offer.
A second example is the triangle named after the mathematician Roger Penrose, the Tribar. It is an "impossible figure" that cannot be built in reality: it shows three bars, each of which seems to be at right angles to the other and at the same time the bars form a triangle. The figure violates several laws of Euclidean geometry; it is as precise as it is unrealizable. Penrose speaks of a "shifting of space", the illusion of space and depth, of a space that is both logical and illogical. A third example is the Möbius loop: If the ends of a ribbon are glued together in such a way that one side is turned by 180°, then it is no longer orientable, i.e. you cannot distinguish between bottom and top or between inside and outside.
Finally, the artist refers to string theory, which assumes that our world is not just made up of small atoms and even smaller particles, often imagined as tiny spheres or dots. But that our reality is made of lines or threads, so called "strings". We have already spoken about the strings in the work. These strings vibrate like the strings of a guitar. And while in everyday life we move in a three-dimensional space - back and forth, sideways, up and down - string theorists assume a dozen or more dimensions and see both space and time as "bent", folded.
Christopher Sage is certainly not interested in illustrating scientific theories and their peculiarities. Rather, his theme is the absurd, fantastic and irrational in the rational as such. Or, to put it very broadly: These works reflect our efforts to give life a "reasonable" meaning - efforts that are doomed to fail because of the unpredictable and contradictory, the chaotic nature of our existence. It is the miracle of life that in the end a lot succeeds nonetheless - just as all the immanent contradictions of these artworks are resolved in their elegant, vibrant, perfect beauty.
One of the central topics of painting is the transformation of three- and four-dimensional reality into the two-dimensional picture plane. This corresponds to the theories on space and time mentioned above. There is no need to talk further about space; the dimension of time is represented by the heavily moving swirls of colour (of course not expressive action painting, but well-calculated brush work) or the flat bands that jump merrily through the narrow picture space, "ping-ponging", as the artist calls it. Such aspects touch on a crucial question of this work: what is art and how is it created?
Whoever tries to produce art according to prefabricated models will fail. The irrational - the emotion - plays the dominant role. Take the golden section, one of the eternal foundations of art. Most artists are certainly able to reproduce this division ratio solely from their sense of form. But it can also be calculated and brought to a mathematical formula - which then, however, leads to a number that is considered transcendent or irrational. So this is a mathematical proof that the basis of beauty is the irrational.
Christopher Sage confronts the reasoned art of Constructivism with the irrational; in his works cool, mathematical reflections hit upon surprising, intuitive, even carnivalesque notions. Symbolic for this is the prime number that dominates large parts of the work: it is as clear as it is mysterious.
These cooly calculated and mathematically desgined works stand in the tradition of Constructivism of the first third of the 20th century: Russian artists like Tatlin or Malevitch and movements such as de Stijl and Bauhaus paved the path. However, the other side is immediately evident as well: There is also the irrational and mystic, the „sur-real“ aspect. His paintings, Christopher Sage says, “are in a kind of neurosis…“ An indication are their bright, „electric“ colors, that stand in sharp contrast to apparently deserted, melancholic scenes.
This tension continues in the subject matters and motifs. There is, for instance, a Fortune Teller – what could be farther away from rationality, characterized by the Constructivist pattern, than predicting one’s future through a crystal ball? Accordingly, Christopher Sage lets color splashes fly around the sphere. Or the triangular construction in Penrose: This figure is as precise as it is unreal, it violates several laws of Euclidean geometry and cannot be built. We are familiar with this kind of structures through the picture puzzles of M. C. Escher, yet one of the inventors of this special „impossible figure“ is the mathematician Roger Penrose, whose Tribar Sage quotes.
Or take the prime numbers on which large parts of this work are based; all the paintings’ dimensions are defined by prime numbers. The prime number is perfectly clear, it is a number that has no positive divisors other than 1 and itself. And yet it is full of mysteries: We are far from knowing their exact quantity, all we know is that the sequence never ends; the most recent result is a number with 22.338.618 decimal places. Also, we do not understand the logic of the placement of prime numbers on the number line. Many think prime numbers are the most beautiful thing math has to offer.
One of the founders of Constructivism was Paul Cézanne who reduced nature to the geometric figures cylinder, sphere and cone; Sage illustrates this in After Cézanne. The Impressionist came to his conclusion after having depicted his motif, the Montagne Sainte-Victoire, over and over again. In the end he was absorbed by this landscape – he disappeared in his motif. The Apprentice depicts the start of the process, when he begins to turn into geometric forms. In other works (The Telekenisist, Ribbon) Sage dissolves heads and abstract forms in swirls of color and brush that stand out effectively against the basic patterns.
Christopher Sage shows us the world as a rational, mathematical construct in which the irrational spreads with quite a stir – just like the pictorial spaces often break and diverse modes of representation collide with each other. To quote Albert Einstein: Mathematical theories about reality are always unsecured – when they are secure, they don’t deal with reality. What counts is intuition.
Christopher Sage’s paintings lure the viewer into a world of abandoned carnivals and puzzling mazes using mathematics, magic, mysticism, and fiction. The range of his works as well as the dimensions and form of the pictorial elements are all defined by prime numbers. His illusionist-like spaces are based on two-point perspective and other mathematical techniques.
After priming the canvas, Sage applies a base layer of paint of muted colors such as beige, dark brown, or green. Then he applies a colorful pattern of triangles, rectangles, rhombus-forms, and other simple mathematical shapes, which first recall wallpaper from the 1960s and 1970s. The ensuing pattern is aligned according to the two vanishing points in the interior of the picture, so that the eye follows the lines of composition along a pathway of shapes and pictorial elements. Sometimes the shapes are mirrored, folded, or rotated, creating spaces of sophisticated visual suspense. What is ceiling, what is floor, which path leads to a new space, which to a dead end? Forms can be superimposed transparently onto others or can be objects that cast shadows. Wooden posts as elements of real exhibition spaces jut through the composition like foreign bodies, so that the geometric fields of color seem like easels or exhibition walls. Zigzag bands, like Ariadne’s thread, run through the picture without allowing viewers to leave the maze. On the contrary — they are constantly forced to question or change their own viewpoint and reorient themselves.

After the show,
2012 35.9 x 36.7cm acrylic paint on canvas on board |

After the show installation view ,
2012 dimensions variarble After the show, wood & bunting |
The deserted spaces in these works become even more unreal when an incongruous piece of furniture appears. These are sometimes absorbed, obscured, and partially covered by areas of color but never disappear entirely. Sage makes use of a wide, at times even conflicting, palette. He defines his fields of color with precision or places a single cube or cone into the composition for spatial emphasis. You would not expect a chain of colorful pennants to show up in this highly mathematical formal language, yet, when it does, you immediately think of an abandoned circus.
Installations
Christopher Sage’s interest in optical illusion began while studying at the University in Reading and led to a series of sculptures, installations, and, later, paintings. It’s like being in a hall of mirrors when the three-dimensional structure is suddenly confronted with the created, two-dimensional image.
Now Sage also conveys this idea of seemingly unrelated elements in his paintings to the exhibition space itself: the pattern on the walls, the easels, dividing walls, pillars, or chains of colorful pennants. Again, arrangement and accoutrement are based on prime numbers.

101 years,
2013 installation dimensions variarble 100 years upside down, The 3, Helix & house-paint |

100 years upside down,
2012 36.7 x 37.3cm acrylic paint on canvas on board |
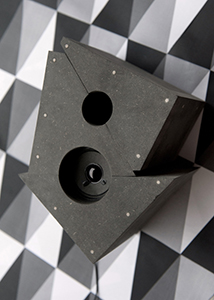
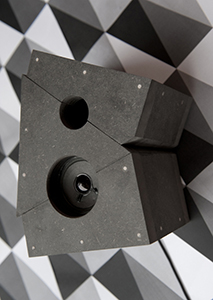
In one of his installations, Sage cuts three identical black loudspeakers into three parts using a ratio of prime numbers and angles. Then he reassembles them, but uses one part of the other two, for each of the three new speakers. This results in the XYZ, ZXY, and YZX black-box resonators. Three very different forms are created from three identical loudspeakers. The length of the audio loop sounding from the speakers is also based on prime numbers, for example, 4min. 01sec. = 241sec.
 |
 |
 |
Works from 2012 and 2013
In 2012 and 2013, Christopher Sage began to merge people into his otherwise mathematical pictorial spaces and the patterns they contained. Sage is interested in how the visual world is enhanced by an historical, intellectual one.
The starting point for the painting “Emile the Great (Paul)” is a photograph taken by the painter Emile Bernard (1868- 1941) of Paul Cezanne (1839-1906) shortly before his death. The portrait and the exchange of letters between the two painters are shaped by Bernard's deep respect for Paul Cezanne, the father of modern painting. Cezanne’s work dealt with the relationship between what we see and what is really there. He wanted to depict what we actually see. For years he painted his favorite motif, the Montagne Sainte-Victoire in Provence, en plein air, again and again. His ever-deepening involvement with this unique landscape was so strong that Sage saw a connection to Douglas Edison Harding (1909-2007) and his essay "On Having No Head,” in which the philosopher, author, and spiritual teacher describes his merging with a landscape during a walk in the mountains. He writes about how a panorama of the surrounding nature took the place of his head. Cezanne seems to have experienced the same phenomenon while paintings.
Sage’s work, “Emile the Great (Paul),” depicts this sense of “dissolving into something else.” In the picture, Cezanne’s upper body towers into the scene like a large, green mountain and merges with the zigzag patterns of the background. Where the head would normally be, there is only an empty, almost magically illuminated mountain peak, with a cylinder hovering above. The title of the work, “Emile the Great (Paul),” refers to a magician who uses magic to make his head vanish.
The counterpart of this work is called “The Apprentice (Paul)” and shows a portrait of the young Paul Cezanne taken from a photo by an unknown photographer. Again, Cezanne has lost his head. The eyes and nose have been replaced by a pyramid and a red circle. If you hang “The Apprentice (Paul)” to the left of “Emile the Great (Paul),” the zigzag stripes continue left to right from one image to the next. Sage’s combination of conceptual, thematic, and painterly components depicts the development of Cezanne from a young painter into a master of his craft.

The Apprentice (Paul),
2013 47 x 41cm oil & acrylic paint on canvas |

Emile the Great (Paul),
2012 47 x 41cm oil & acrylic paint on canvas |
Christopher Sage’s work becomes the stage for different ideas. Numbers, their interrelationship, historical circumstances, and his own thoughts give new identity to the two-dimensional pictorial language and allow for many different interpretations — hence the transfer from the two-dimensional into the three-dimensional world.